Homology theory: Difference between revisions

This redirect is about the homology of topological spaces. For the homology of other mathematical objects, see Homological algebra.

In mathematics, homology theory is the axiomatic study of the intuitive geometric idea of homology of cycles on topological spaces.

General idea

A torus with generators colored in pink and red.

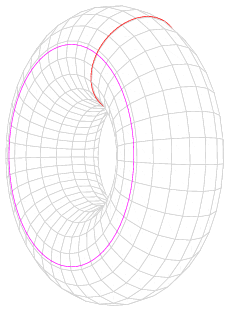
To any topological space $X$ and any natural number $k$, one can associate a set $H_{k}(X)$, whose elements are called ($k$-dimensional) homology classes. There is a well-defined way to add and subtract homology classes, which makes $H_{k}(X)$ into an abelian group, called the $k$th homology group of $X$. In heuristic terms, the size and structure of $H_{k}(X)$ gives information about the number of $k$-dimensional holes in $X$. For example, if $X$ is a figure eight, then it has two holes, which in this context count as being one-dimensional. The corresponding homology group $H_{1}(X)$ can be identified with the group $\mathbb {Z} \oplus \mathbb {Z}$ of pairs of integers, with one copy of $\mathbb {Z}$ for each hole. While it seems very straightforward to say that $X$ has two holes, it is surprisingly hard to formulate this in a mathematically rigorous way; this is a central purpose of homology theory.

For a more intricate example, if $Y$ is a Klein bottle then $H_{1}(Y)$ can be identified with $\mathbb {Z} \oplus \mathbb {Z} /2\mathbb {Z}$. This is not just a sum of copies of $\mathbb {Z}$, so it gives more subtle information than just a count of holes.

The formal definition of $H_{1}(X)$ can be sketched as follows. The elements of $H_{1}(X)$ are one-dimensional cycles, except that two cycles are considered to represent the same element if they are homologous. The simplest kind of one-dimensional cycles are just closed curves in $X$, which could consist of one or more loops. If a closed curve $C_{0}$ can be deformed continuously within $X$ to another closed curve $C_{1}$, then $C_{0}$ and $C_{1}$ are homologous and so determine the same element of $H_{1}(X)$. This captures the main geometric idea, but the full definition is somewhat more complex. For details, see singular homology. There is also a version (called simplicial homology) that works when $X$ is presented as a simplicial complex; this is smaller and easier to understand, but technically less flexible.

For example, let $T$ be a torus, as shown on the right. Let $C$ be the pink curve, and let $D$ be the red one. For integers $n$ and $m$, we have another closed curve that goes $n$ times around $C$ and then $m$ times around $D$; this is denoted by $nC+mD$. It can be shown that any closed curve in $T$ is homologous to $nC+mD$ for some $n$ and $m$, and thus that $H_{1}(T)$ is again isomorphic to $\mathbb {Z} \oplus \mathbb {Z}$.

Cohomology

As well as the homology groups $H_{k}(X)$, one can define cohomology groups $H^{k}(X)$. In the common case where each group $H_{k}(X)$ is isomorphic to $\mathbb {Z} ^{r_{k}}$ for some $r_{k}\in \mathbb {N}$, we just have $H^{k}(X)=Hom(H_{k}(X),\mathbb {Z} )$, which is again isomorphic to $\mathbb {Z} ^{r_{k}}$, and $H_{k}(X)=Hom(H^{k}(X),\mathbb {Z} )$, so $H_{k}(X)$ and $H^{k}(X)$ determine each other. In general, the relationship between $H_{k}(X)$ and $H^{k}(X)$ is only a little more complicated, and is controlled by the universal coefficient theorem. The main advantage of cohomology over homology is that it has a natural ring structure: there is a way to multiply an $i$-dimensional cohomology class by a $j$-dimensional cohomology class to get an $i+j$-dimensional cohomology class.

Applications

Notable theorems proved using homology include the following:

• The Brouwer fixed point theorem: If $f$ is any continuous map from the ball $B^{n}$ to itself, then there is a fixed point $a\in B^{n}$ with $f(a)=a$.
• Invariance of domain: If U is an open subset of $\mathbb {R} ^{n}$ and $f:U\rightarrow \mathbb {R} ^{n}$ is an injective continuous map, then $V=f(U)$ is open and $f$ is a homeomorphism between $U$ and $V$.
• The Hairy ball theorem: any vector field on the 2-sphere (or more generally, the $2k$-sphere for any $k\geq 1$) vanishes at some point.
• The Borsuk–Ulam theorem: any continuous function from an n-sphere into Euclidean n-space maps some pair of antipodal points to the same point. (Two points on a sphere are called antipodal if they are in exactly opposite directions from the sphere's center.)

Intersection theory and Poincaré duality

Let $M$ be a compact oriented manifold of dimension $n$. The Poincaré duality theorem gives a natural isomorphism $H^{k}(M)\simeq H_{n-k}(M)$, which we can use to transfer the ring structure from cohomology to homology. For any compact oriented submanifold $N\subseteq M$ of dimension $d$, one can define a so-called fundamental class $[N]\in H_{d}(M)\simeq H^{n-d}(M)$. If $L$ is another compact oriented submanifold which meets $N$ transversely, it works out that $[L][N]=[L\cap N]$. In many cases the group $H_{d}(M)$ will have a basis consisting of fundamental classes of submanifolds, in which case the product rule $[L][N]=[L\cap N]$ gives a very clear geometric picture of the ring structure.

Connection with integration

Suppose that $X$ is an open subset of the complex plane, that $f(z)$ is a holomorphic function on $X$, and that $C$ is a closed curve in $X$. There is then a standard way to define the contour integral $\oint _{C}f(z)dz$, which is a central idea in complex analysis. One formulation of Cauchy's integral theorem is as follows: if $C_{0}$ and $C_{1}$ are homologous, then $\oint _{C_{0}}f(z)dz=\oint _{C_{1}}f(z)dz$. (Many authors consider only the case where $X$ is simply connected, in which case every closed curve is homologous to the empty curve and so $\oint _{C}f(z)dz=0$.) This means that we can make sense of $\oint _{c}f(z)dz$ when $c$ is merely a homology class, or in other words an element of $H_{1}(X)$. It is also important that in the case where $f(z)$ is the derivative of another function $g(z)$, we always have $\oint _{C}g'(z)dz=0$ (even when $C$ is not homologous to zero).

This is the simplest case of a much more general relationship between homology and integration, which is most efficiently formulated in terms of differential forms and de Rham cohomology. To explain this briefly, suppose that $X$ is an open subset of $\mathbb {R} ^{N}$, or more generally, that $X$ is a manifold. One can then define objects called $n$-forms on $X$. If $X$ is open in $\mathbb {R} ^{3}$, then the 0-forms are just the scalar fields, the 1-forms are the vector fields, the 2-forms are the same as the 1-forms, and the 3-forms are the same as the 0-forms. There is also a kind of differentiation operation called the exterior derivative: if $\alpha$ is an $n$-form, then the exterior derivative is an $(n+1)$-form denoted by $d\alpha$. The standard operators div, grad and curl from vector calculus can be seen as special cases of this. There is a procedure for integrating an $n$-form $\alpha$ over an $n$-cycle $C$ to get a number $\oint _{C}\alpha$. It can be shown that $\oint _{C}d\beta =0$ for any $(n-1)$-form $\beta$, and that $\oint _{C}\alpha$ depends only on the homology class of $C$, provided that $d\alpha =0$. The classical Stokes's Theorem and Divergence Theorem can be seen as special cases of this.

We say that $\alpha$ is closed if $d\alpha =0$, and exact if $\alpha =d\beta$ for some $\beta$. It can be shown that $dd\beta$ is always zero, so that exact forms are always closed. The de Rham cohomology group $H_{dR}^{k}(X)$ is the quotient of the group of closed forms by the subgroup of exact forms. It follows from the above that there is a well-defined pairing $H_{k}(X)\times H_{dR}^{k}(X)\to \mathbb {R}$ given by integration.

Axiomatics and generalised homology

There are various ways to define cohomology groups (for example singular cohomology, Čech cohomology, Alexander–Spanier cohomology or Sheaf cohomology). These give different answers for some exotic spaces, but there is a large class of spaces on which they all agree. This is most easily understood axiomatically: there is a list of properties known as the Eilenberg–Steenrod axioms, and any two constructions that share those properties will agree at least on all finite CW complexes, for example.

One of the axioms is the so-called dimension axiom: if $P$ is a single point, then $H_{n}(P)=0$ for all $n\neq 0$, and $H_{0}(P)=\mathbb {\mathbb {Z} }$. We can generalise slightly by allowing an arbitrary abelian group $A$ in dimension zero, but still insisting that the groups in nonzero dimension are trivial. It turns out that there is again an essentially unique system of groups satisfying these axioms, which are denoted by $H_{*}(X;A)$. In the common case where each group $H_{k}(X)$ is isomorphic to $\mathbb {Z} ^{r_{k}}$ for some $r_{k}\in \mathbb {N}$, we just have $H_{k}(X;A)=A^{r_{k}}$. In general, the relationship between $H_{k}(X)$ and $H_{k}(X;A)$ is only a little more complicated, and is again controlled by the Universal coefficient theorem.

More significantly, we can drop the dimension axiom altogether. There are a number of different ways to define groups satisfying all the other axioms, including the following:

• The stable homotopy groups $\pi _{k}^{S}(X)$
• Various different flavours of cobordism groups: $MO_{*}(X)$, $MSO_{*}(X)$, $MU_{*}(X)$ and so on. The last of these (known as complex cobordism) is especially important, because of the link with formal group theory via a theorem of Daniel Quillen.
• Various different flavours of K-theory: $KO_{*}(X)$ (real periodic K-theory), $kO_{*}(X)$ (real connective), $KU_{*}(X)$ (complex periodic), $kU_{*}(X)$ (complex connective) and so on.
• Brown–Peterson homology, Morava K-theory, Morava E-theory, and other theories defined using the algebra of formal groups.
• Various flavours of elliptic homology

These are called generalised homology theories; they carry much richer information than ordinary homology, but are often harder to compute. Their study is tightly linked (via the Brown representability theorem) to stable homotopy.

Homological algebra and homology of other objects

A chain complex consists of groups $C_{i}$ (for all $i\in \mathbb {Z}$) and homomorphisms $d:C_{i}\to C_{i-1}$ satisfying $dd=0$. This condition shows that the groups $B_{i}={\text{image}}(d:C_{i+1}\to C_{i})$ are contained in the groups $Z_{i}={\text{ker}}(d:C_{i}\to C_{i-1})$, so one can form the quotient groups $H_{i}=Z_{i}/B_{i}$, which are called the homology groups of the original complex. There is a similar theory of cochain complexes, consisting of groups $C^{i}$ and homomorphisms $\delta :C^{i}\to C^{i+1}$. The simplicial, singular, Čech and Alexander–Spanier groups are all defined by first constructing a chain complex or cochain complex, and then taking its homology. Thus, a substantial part of the work in setting up these groups involves the general theory of chain and cochain complexes, which is known as homological algebra.

One can also associate (co)chain complexes to a wide variety of other mathematical objects, and then take their (co)homology. For example, there are cohomology modules for groups, Lie algebras and so on.

References

• Hilton, Peter (1988), "A Brief, Subjective History of Homology and Homotopy Theory in This Century", Mathematics Magazine, 60 (5): 282–291, JSTOR 2689545